Below is the online edition of In the Beginning: Compelling Evidence for Creation and the Flood,
by Dr. Walt Brown. Copyright © Center for Scientific Creation. All rights reserved.
Click here to order the hardbound 8th edition (2008) and other materials.
Changes in the Moon’s Orbit
The Moon was also orbiting the Earth at this same distance (395,884 km) with a velocity of 1.0247 km/sec, found in Equation 4. The Moon was assumed to be in a circular orbit, so there was no radial velocity. However, the Moon did not just move eastward. Because the Moon is inclined relative to the Earth’s equator between 18.28° and 28.58°, its orbit is tipped on average 23.43° relative to the equator (or out of the page if the Moon were shown in Figure 261).1 Therefore, the Moon would have been moving eastward with a velocity of 0.9402 km/sec on average.
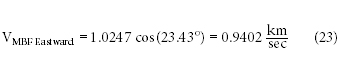
Due to its inclination, the Moon would also have had an average velocity component in the northern or southern direction equal to 1.0247 sin (23.43°) = 0.4074 km/sec as it crossed the equator.
Comparing Equations 22 and 23, notice the Moon would have been moving much faster eastwardly than the debris (0.9402 > 0.007386). This means the Moon would have run into the debris, similar to what would happen if a few boulders were softly tossed in front of a fast moving car. These extremely large rocks reduced the Moon’s energy, which would have dropped the Moon into a lower orbit and decreased its period. It is relatively easy to calculate exactly how the Moon’s velocity would have been changed by the debris. Once the debris entered the Moon’s Sphere of Influence (SOI), it is fair to assume that only the Moon affected the debris’ orbit. Also, because gravity is a conservative force, it is not necessary to determine the exact orbit of the debris inside the Moon’s SOI. All that is needed is to compare the total momentum of the debris and Moon immediately before the debris entered the Moon’s SOI and set this equal to the momentum of the Moon after impact.
Instead of using momentum as the product of mass and velocity (mV), these calculations use µV, which is more convenient and slightly more accurate. (Remember µ is the gravitational constant, G, times the mass of a body.) The following calculations also use the assumptions listed previously that 3% of the Earth’s mass was lost at the time of the flood, and 1.22% of this mass impacted the Moon. Therefore,
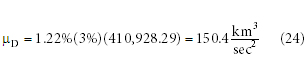
Also, using µ from Equation 1, the gravitational parameter of the slightly heavier Moon after impact would be
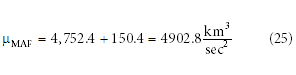
Using these values for µ, the velocity of the Moon after the flood was found in Equation 26 to be 0.04605 km/sec in the radial direction.
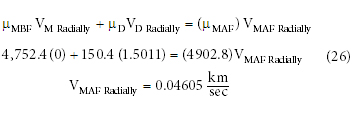
In the eastern direction the Moon would have been moving 0.91158 km/sec after impact.

The Moon would have also been moving in the northern (or southern) direction 0.39503 km/sec after impact.

Using the Pythagorean Theorem, the magnitude of the Moon’s velocity after impact was calculated from these three components to be 0.99454 km/sec.
To summarize, three things changed for the Earth and Moon at the time of the flood that affected the Moon’s orbit:
1. The Earth lost 3% of its mass, so
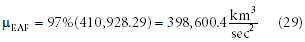
2. The Moon’s mass changed very slightly when 1.22% of the mass ejected from the Earth hit the Moon. As calculated in Equation 25, µMAF = 4902.8 km/sec.
3. The Moon’s velocity changed as found in Equations 26 through 28.
As mentioned previously, when calculating the orbit for large objects like the Moon, the gravitational parameters of the two bodies need to be combined. Therefore,
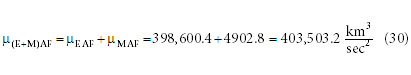
The steps to calculate the semimajor axis and eccentricity of the Moon after the flood follow the exact same process outlined in Equations 10 through 14. First, knowing the new velocity of the Moon, and assuming its position did not change immediately (R = 395,884 km from Equation 3), the Moon’s specific mechanical energy after the flood would be
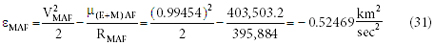
This allows the semimajor axis of the Moon after the flood to be found.
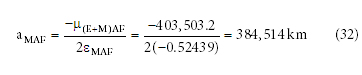
The specific angular momentum of the Moon after the flood is simply the distance the Moon was from the center of the Earth times the velocity in the horizontal direction. In this case, the horizontal velocity of the Moon had an eastwardly and northerly (or southerly) component found in Equations 27 and 28. Using the Pythagorean Theorem, the total horizontal velocity was found.
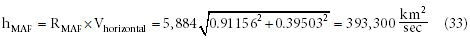
The parameter, p, for the Moon’s orbit after the flood was then found.
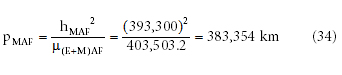
Because p = a (1 - e 2), the eccentricity of the Moon’s orbit after the flood was

From these values the Moon’s radius of perigee, RP , and radius of apogee, RA, after the flood were calculated.
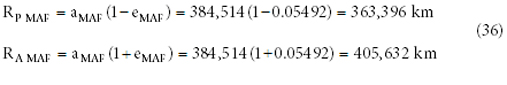
Also, the Moon’s sidereal period can be found.
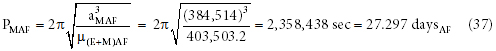
As explained previously, this is the time for the Moon to travel 360° around the Earth. However, the Moon must travel slightly farther between successive full Moons. Prior to the flood, the Moon had to move an extra 30° for each lunar cycle. [See Figure 260.] Because the length of a lunar month today is 29.53 days, and the length of a year is 365.242 days, the Moon must now move an extra 29.106°  today. Therefore, the Moon’s synodic period after the flood should be
today. Therefore, the Moon’s synodic period after the flood should be
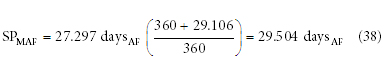
It is actually 29.53 days, which means these calculations are off by only 0.026 days or 37 minutes, with a percent error of only 0.09%. Six other parameters describing the Moon’s orbit around the Earth are even closer to the actual values. All seven of these numbers are summarized in Table 43.